What do we know (and not know) about confinement?
A journey into the confinement phenomenon and the depths of matter
by Nelson Lachini (EuroPLEx fellow, University of Edinburgh)
Have you ever wondered what is inside an atom? We started our journey into the mysteries of matter in 1897 when Joseph Thomson discovered the electron. A decade later, Ernest Rutherford measured unexpected patterns from particle collisions leading to the proposal of a tiny but very massive structure at the heart of the atom: the nucleus. After another decade, Rutherford himself observed the proton as the subatomic particle giving the nucleus its positive electric charge. The proton was the frontline of a cascade of discoveries throughout the 20th century: the neutron, the pion, the kaon, and hundreds of other particles. These are all called hadrons. 
Artistic representation of the internal proton structure (source: Brookhaven National Laboratory).
The search for elementary objects has been the philosophy of particle physics since its early days. However, there is an intricate phenomenon that makes it very challenging for us to understand the world beyond a quadrillionth of a meter: that phenomenon is called confinement, and it is important for at least two reasons. Without confinement, matter in the universe simply would not exist as we know it. But moreover, the inner workings of confinement have been a mystery that has kept physicists and mathematicians busy since… There is even a million-dollar prize to whoever demonstrates it mathematically.
Suppose we try to apply Rutherford’s method to hadrons. After preparing a very delicate experimental apparatus, we finally manage to collide, for example, two protons. What we see is everything but simple; a myriad of hadrons and other particles, such as electrons and muons, are ejected out from the collision point, but we cannot seem to spot suitable candidates for the constituent objects of the initial protons. In the 1970s, the theory of Quantum Chromodynamics (QCD) proposed that hadrons are composed of even tinier particles called quarks and gluons. These would always conspire to not be identified; somehow, they were confined inside the hadrons.

GlueX experiment at JLAB (source: Wikipedia).
The confinement phenomenon is part of a discovery process that is gradual and interdisciplinary. Nowadays, with the data from collider experiments and the theoretical predictions from computer simulations, we have conclusive evidence that confinement is governed by QCD and we know its basic mechanisms. Somewhat distant but not apart, mathematicians still try to show how this concept can be rigorously defined. Confinement is one of the central concepts of particle physics and is of ultimate importance to the understanding of subnuclear interactions, besides still being an open problem in pure mathematics.
The colliders
Particle physics enthusiasts probably know that the Standard Model, and the possibility of finding physics beyond it, motivate the large collider experiments, starring the famous Large Hadron Collider (LHC) at CERN, followed by many others, such as the GlueX experiment at JLab. The Standard Model successfully describes a great number of phenomena observed at those experiments over a fair range of energies, involving the strong, weak and electromagnetic forces. However, in the billions and billions of events catalogued in colliders, we were never able to capture a quark, only hadrons and many other “quarkless” particles.
Of course, you could wonder how we even know that quarks are actually there and that confinement is not just a bad excuse for their non-existence. Indeed, around 1970, before the establishment of QCD, the quark model was viewed as a mathematical image of what was going on inside pions and other hadrons, but not as a direct description in terms of physical objects. But it is not necessary to delve into the philosophical questions of modern physics here: quarks were simply not taken seriously by the scientific community.
Soon enough QCD was born, putting together quarks and gluons in the consistent framework of gauge theories. The understanding of quantum field theory at the time predicted that quarks could be isolated in particle colliders; but many years have passed, and today we have overwhelming experimental evidence that QCD works and still no direct detection of quarks was made.
String breaking example: pair of quark-antiquark breaks into another pair (created by N. Lachini).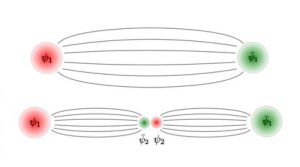
One of the most conspicuous mechanisms of confinement is called “string breaking”, the snapping of the chromoeletromagnetic tube that binds the quarks together. To understand this process, we can imagine the bond between a quark-antiquark pair as a sturdy “string” (this kind of hadron is called a meson). To have an idea of the magnitude, such a string could hold a few cars by stretching out a billionth of a meter. If we want to separate the quark from the antiquark, we have to apply energy to the system as if the string was being pulled out. However, at a certain point, the energy is so large that it allows the creation of a new quark-antiquark pair (the energy-mass equivalence: E=mc2). This happens at such small distances that it is impossible to resolve the constituent quark or antiquark at colliders. What happens often is that a chain of string fragmentations is triggered and a hadron jet is observed at the detector. Jet physics is a well-established subfield of particle physics whose basic principles stem from string breaking. This kind of well-known phenomenon gives valuable phenomenological support to confinement.
The supercomputers
Besides experimental tests, strong evidence showing that confinement emerges from QCD come also from a central subject field of EuroPLEx: lattice QCD. In 1974, Kenneth G. Wilson in his paper “Confinement of Quarks” developed a way to assess confinement from a theoretical point of view, through a quantity that today we call “Wilson loop”. In the same paper, Wilson also gave birth to Lattice Gauge Theory, and from there it became possible to simulate QCD on a computer.
When we put QCD on a finite lattice, the theory gets discretized and becomes well-defined. Furthermore, the methods of lattice QCD in its majority do not assume that the quantum fields are weakly excited. This gives us access to phenomena where the fields can interact strongly. In particular, a showcase example is the spectrum of hadron masses obtained from lattice QCD.
Taking Wilson’s work as a starting point, lattice QCD foundations were developed in parallel with the increase of computational power across the world. Until the 1990s, it was very difficult to simulate QCD considering the interaction of quarks that pop in and out from the quantum vacuum and, consequently, the simulations predicted the hadron spectrum with fairly uncontrolled statistical errors.
In the 2000s, with the advance of supercomputers and new algorithms, theoretical physicists performed the first realistic simulations of QCD with dynamical quarks on the spectrum of hadrons: masses simulated from lattice QCD (red points) matched very well their experimental measurements (dark lines).
The way hadrons acquire their masses is due to an effect called “dynamical mass generation”, which is a non-trivial mechanism correlated to confinement. This is an example of confirmation that makes physicists very confident that confinement arises from QCD. On top of that, through lattice QCD simulations it is possible to calculate the energy between a quark and antiquark and observe that it raises linearly with the distance between them. Such linear behaviour is characteristic of a string being stretched out and is a good indicator of confinement, as we have seen. Simulations with dynamical quarks go even further and also show us that QCD gives rise to string breaking, which is another positive evidence for confinement.
Another notion of confinement is that of colour confinement. QCD is governed by the interchange of colour charge (so the suffix chromo), analogous to the electrical charge in electromagnetism. In QCD, gluons and quarks carry colour charge and we say they are coloured. Colour confinement entails that not only quarks but any coloured state cannot be observed at detectors. That includes gluons or any composite particle that is not colour neutral.
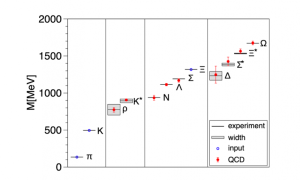
The light hadron spectrum of QCD (source: https://arxiv.org/pdf/0906.3599.pdf).
The search for particles composed only of gluons, in experiments and simulations, is another research subbranch that helps pin down the workings of confinement. These particles, called glueballs, are massive bound states predicted by lattice simulations since the 1980s. This kind of particle is theoretically possible to be detected but its mixing with hadrons imposes practical difficulties to experimentalists. Recently, experiments have been reporting strong signals that for example the odderon, a glueball with an odd number of gluons, is being observed at the LHC and Tevatron colliders. Note that gluons do not have any mass; the glueball is a state of pure strong force stored in the chromoeletromagnetic tubes keeping the constituent gluons together.
On top of this extensive numerical and experimental evidence, many researchers try to model confinement from different perspectives: dual superconductivity, monopoles, centre vortices, and others. These are successful pictures of confinement to some extent, in the sense that they can reproduce several of its characteristics, such as string breaking. Note that these models are not directly connected to QCD, but are attempting to describe confinement through effective mechanisms, using both simulations and analytical calculations. It is hoped that in the future we will be able to trace them back to QCD and explain how they emerge from it, giving us a better theoretical understanding of this contrived phenomenon.
The prize
Until now we have contemplated the “physicist view” of confinement. Another way we can think about it is through pure mathematics. In 2000, the Clay Mathematics Institute established the seven Millennium Prize Problems with the help of specialists in mathematics and theoretical physics. One of those famous seven problems is related to confinement: if one discovered how it is rigorously derived from QCD, one would probably become a millionaire, after some years of validation by peers. Until now, just one of the seven problems, the so-called “Poincarè conjecture“, has been solved. It was cracked by the Russian mathematician Grigori Perelman in 2003 and recognized by the Clay Institute in 2006. Curiously, Perelman refused the prize.
The Millennium Problem related to confinement goes by the formal name of “Yang–Mills existence and mass gap” problem. To be honest, confinement is only a part of the problem: one would also need to explain the inexistence of massless physical states (this is what the “mass gap” in the name refers to), as well as chiral symmetry breaking, a phenomenon that, together with confinement, describes the masses of hadrons through the breaking of fundamental symmetries in QCD. It is believed these three questions are intimately related so it is unlikely that someone proves only one of them knowing nothing about the other two.
However, the deal is that we are talking about a specific type of mathematical “explanation”: propose a set of axioms and prove their consequences. Even though we can say a lot about quantum field theories on continuum spacetimes at various levels of mathematical rigor, we have not axiomatically formulated realistic QCD or its version without quarks, which are the steps required for winning the prize. Of course, this does not prevent us from making very concrete predictions using QCD and comparing it to experimental results – most probably mathematics is still catching up with physics, as frequently happened in history.
It all started with the proposal and the empirical observation that quarks could not be captured individually by colliding hadrons. The advance of computational power then gave us the theoretical evidence that QCD not only proposes the quarks and gluons, but also predicts their elusiveness. Confinement is an extremely rich phenomenon, with a central role in the description of strong interactions and gives rise to questions in theoretical and experimental physics, as well as in pure mathematics.
Clearly, the type of questions asked in physics is not the same as the ones asked in mathematics. Rigorously demonstrating that a theory exists is immediately important to mathematicians, but physicists have a high level of confidence that QCD and confinement work at the level of the high-precision tests done at particle colliders and simulations. Nevertheless, the scientific community would gladly welcome a mathematical proof of confinement for it would likely come with a set of tools useful to the advance of physics beyond the Standard Model.
Sources:
– https://en.wikipedia.org/wiki/GlueX (Accessed on 05/04/2021)
– https://arxiv.org/pdf/0906.3599.pdf (Accessed on 23/05/2021)
– https://cerncourier.com/a/odderon-discovered/ (28/06/2021)
– http://www.claymath.org/millennium-problems/yang%E2%80%93mills-and-mass-gap (Accessed on 05/04/2021)
– https://www.theguardian.com/world/2010/mar/23/grigory-perelman-rejects-1m-dollars
Original story translated with permission from Investigación y Ciencia.

